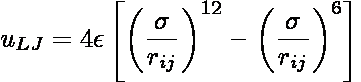Benchmark results for the Stockmayer fluid

This website is best viewed with younger browsers (for example: Netscape 7, IE 6, Opera 7, Firefox 1)

The purpose of these pages is to provide some explicit results from Monte Carlo simulations for the Stockmayer fluid. It is intended to provide guides for testing codes. Reproducing these results is a test of the correctness of codes, either written by the user or obtained elsewhere. The explicit conditions for each of the sets of results are supplied so that meaningful comparisons of your results with the ones listed here are possible.

The information presented here has been organized into several different pages. It is available as web pages or as a single excel file (not yet available).

  1. Saturation Properties: Monte Carlo results for the saturated liquid and vapor at several temperatures for various dipole strengths.
  2. Equation of State: pressure as a function density at various temperatures and dipole strengths.

 

In the Stockmayer fluid, particles interact through the following pairwise additive potential

u = uLJ + ud

where uLJ is the familiar Lennard-Jones potential

           .

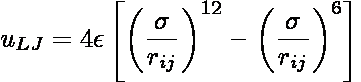and ud dipolar interaction between point dipoles embedded at each particle center

      .

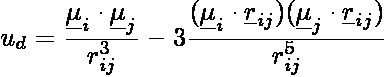In the above equations, rij is the separation vector between particles i and j with a magnitude rij and  �k is the dipole moment of particle k.

The Ewald summation is used to deal with the slowly-decaying but infinitely long ranged nature of the electrostatic electrostatic interaction. Perfectly conducting boundary conditions are used. The reader is referred to Ref [1].

 

 

This is a work in progress. Additional properties and dipole strengths will be added as they become available.

References

[1] M. P. Allen and D. J. Tildesley, Computer Simulation of Liquids (Oxford University Press, New York, 1989).